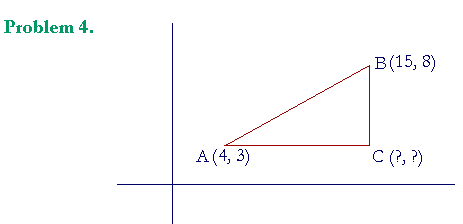31
RECTANGULAR COORDINATES
Actual versus potential infinities
WE WILL BEGIN with vocabulary.
First, a coordinate. A coordinate is a number that labels a point on a line.
The coordinate 0 is called the origin of coordinates. Points to the right of 0 are labeled with positive numbers: 1, 2, 3, etc. Points to the left of the origin are labeled with negative numbers: −1, −2, −3, etc. . Those coordinates are the "addresses" of those points.
A coordinate axis is a line with coordinates.
Now, to label the points in a plane, we will need more than one coordinate axis, and we place a second at right angles to the first.
Points above the origin have positive coordinates; points below have negative coordinates.
Those lines are called rectangular coordinate axes, because they are at right angles to one another; the coordinates on them are called rectangular coordinates. They are also called Cartesian coordinates, after the 17th century philosopher and mathematician René Descartes; for he was one of the first to realize the possibility of solving problems of geometry by means of algebra with the coordinates. Hence we have the name coordinate geometry or, as it is often called, analytic geometry.
The rectangular coordinates of a point are an ordered pair, (x, y).
The pair (2, 3) -- over 2 and up 3 -- labels a different point than (3, 2): over 3 and up 2. The horizontal coordinate -- Right or left -- is always entered first. The vertical coordinate -- Up or down -- is always entered second. For that reason, (2, 3) is called an ordered pair.
The coordinates of the origin are (0, 0). We don't move right or left and we don't move up or down. We will see that 0 is an extremely important coordinate. It means that the point is on one of the axes.
Now the horizontal axis is always called the x-axis, and the vertical axis is always called the y-axis. The first coordinate, then, is called the x-coordinate; the second is called the y-coordinate. We always write (x, y).
Finally, the coordinate axes divide the plane into four quadrants:
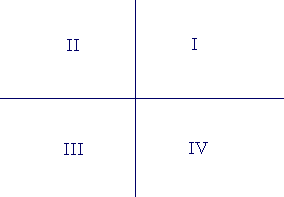
The first, the second, the third, and the fourth. The quadrants are labeled counter-clockwise.
Problem 1. Name the coordinates of each point.
To see the answer, pass your mouse over the colored area.
To cover the answer again, click "Refresh" ("Reload").
Do the problem yourself first!
| a) |  |
b) |  |
c) |  |
||||||||
| (2, 3) | (3, 2) | (−1, 3) | |||||||||||
| d) |  |
e) |  |
f) |  |
||||||||
| (3, −1) | (−2, −1) | (0, 3) | |||||||||||
| g) |  |
h) |  |
i) |  |
||||||||
| (3, 0) | (−2, 0) | (0, −2) | |||||||||||
Problem 2. Coordinate 0.
a) On the x-axis, what is the value of every y-coordinate? 0
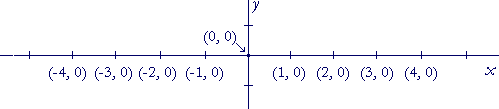
On the x-axis, we don't move up or down. At every point, y = 0.
b) On the y-axis, what is the value of every x-coordinate? 0
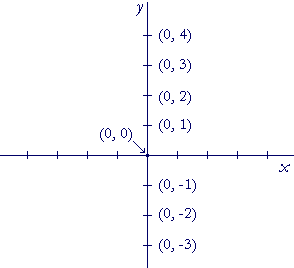
On the y-axis, we don't move right or left. At every point, x = 0.
c) Where is the y-coordinate always 0? On the x-axis.
d) Where is the x-coordinate always 0? On the y-axis.
Problem 3.
a) Where is the x-coordinate always 2?
On the vertical line 2 units to the right of the origin.
In fact, we say that that vertical line is the graph of the equation x = 2 – on every point of that line, the x-coordinate is 2 – and that x = 2 is the equation of that line.
b) Where is the y-coordinate always −3?
On the horizontal line 3 units below the origin.
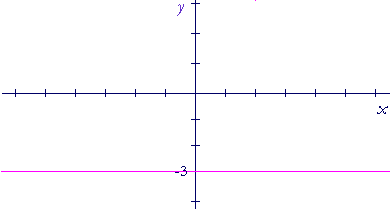
That line is called the graph of y = −3. And y = −3 is called the equation of that line.
Problem 4. In which quadrant does each point lie? Or is it on an axis; if so, which axis?
| a) | (2, −3) Fourth | b) | (−4, 2) Second | |
| c) | (0, −5) On the y-axis. | d) | (−3, −1) Third | |
| e) | (5, 0) On the x-axis. | f) | (−6, 9) Second | |
| g) | (0, −4) On the y-axis. | h) | (−4, 0) On the x-axis. | |
| i) | (−1, −1) Third | j) | (0, 6) On the y-axis. | |
| k) | (−1, 0) On the x-axis. | l) | (0, 1) On the y-axis. | |
| m) | (5, −2) Fourth | n) | (−5, 0) On the x-axis. | |
The extremities of a straight line AB have coordinates (4, 3) and (15, 8), and that line is the hypotenuse of a right triangle ABC.
Name the coordinates of the right angle at C.
C has the same x-coordinate as B. Therefore its x-coordinate is 15. And C has the same y-coordinate as A. Therefore its y-coordinate is 3. The coordinates at C are (15, 3).
Actual versus potential infinities
The idea of an actually infinite straight line is that it has no extremities
-- no endpoints.
Obviously, that can be only an idea, because it is impossible to draw one
A potentially infinite straight line, on the other hand, has two extremities.
It is potentially infinite in the sense that we may extend it in either direction for as far as we please. It is a line that we could actually draw.
The student should be warned that when writers use the expression "straight line" these days, they invariably mean an actually infinite line. Hence, they refer to any finite line, with its two extremities, as a line segment. They imagine that every finite straight line is a segment of an actually infinite line. That has been the authoritative point of view since the late eighteenth century, when it also became authoritative to say that the real numbers stretched continuously from "minus infinity to infinity." Therefore the x-axis -- that "straight line" -- also had to be actually infinite.
In classical plane geometry, however, lines are potentially infinite. Only what we have actually drawn, that is, actually produced, can we say mathematically exists. That is the essence of the logical principle that we may not assume that just because something has been defined, it exists. For if we automatically accept every idea, then we have entered the realm of fantasy mathematics.
See First Principles of Euclidean Geometry, Commentary on the Definitions.
A ray is the idea of a straight line with one extremity.
Next Lesson: The Pythagorean distance formula
Please make a donation to keep TheMathPage online.
Even $1 will help.
Copyright © 2001-2010 Lawrence Spector
Questions or comments?
E-mail: themathpage@nyc.rr.com