skill

S k i l l
 i n
A R I T H M E T I C

Table of Contents | Home | Introduction

Lesson 20  Section 2

Comparing fractions

Back to Section 1
 10.   What is the relative size of fractions that have equal numerators?
fractions
  The larger the denominator, the smaller the fraction.
 

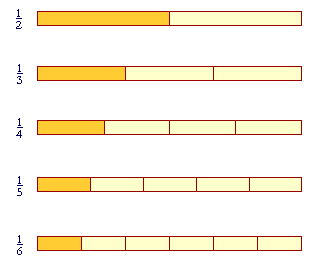
fractions

Those fractions are getting smaller.  As the denominator -- the number of equal parts -- gets larger, then the size of each part gets

  smaller.   1
6		  is smaller than  1
5		 .

Also, since one-sixth is smaller than one-fifth, then two will be smaller than two:

2
6		  is smaller than  2
5		 .

Three will be smaller than three:

3
6		  is smaller than  3
5		 .

And so on.

When frations have equal numerators, then the larger the denominator,
the smaller the fraction.

2
3		 ,  2
4		 ,  2
5		 ,  2
6		 .

Those fractions are getting smaller.


In terms of ratios, the ratio of 1 to 2, for example, is greater than the ratio of 1 to 3:

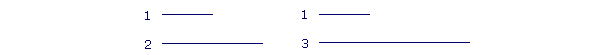
fractions

When we compare 1 with 2, it appears greater than when we compare it with 3.
 11.   What is the relative size of fractions that have equal denominators?
fractions
 
  The larger the numerator, the larger the fraction.
 

In this sequence,

2
6		 ,  3
6		 ,  4
6		 ,  5
6		 ,

the fractions are getting larger.  Each one is one more of the 6 equal parts into which number 1 has been divided.

As for ratios, we say that the ratio of 2 to 5 is smaller than the ratio of 3 to 5:

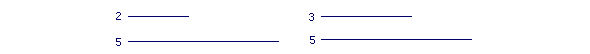
fractions

2, when compared with 5, appears smaller than 3 when compared with 5.

Example 1.   Arrange these from smallest to largest:

5
7		   4
9		   4
7
  Solution.  We must compare them in pairs.   4
9		  and  4
7		  have the same 

numerator; therefore

4
9		  is smaller than  4
7		 .
5
7		 and 4
7		 have the same denominator; therefore
5
7		  is larger than  4
7		 .

The sequence is

4
9		   4
7		   5
7		 .

  Example 2.   Which is smaller,    1 
10		  or  2
9		 ?
  Answer.  Since   1 
10		  is smaller than  1
9		 , then it is surely smaller than  2
9		 .

In Lesson 23 we will see how to compare fractions with different numerators and denominators.


Please "turn" the page and do some Problems.

or

Continue on to the Section 3.

Section 1 on Fractions

Introduction | Home | Table of Contents

Copyright © 2021 Lawrence Spector

Questions or comments?

E-mail:  teacher@themathpage.com