Table of Contents | Introduction | Home
![]()
P l a n e G e o m e t r y
An Adventure in Language and Logic
based on

THE SIDES AND ANGLES OF A TRIANGLE
Book I. Proposition 20
Here, finally, is Proposition 20. It depends on Proposition 18. To prove, in triangle ABC, that sides BA, AC are together greater than side BC, on side AC we construct the isosceles triangle DAC.
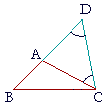
Since AC is equal to AD, then side BD -- which is BA, AD -- is equal to BA, AC. It is not difficult to see that angle DCB is greater than angle D, so that the opposite side BD is greater than opposite side BC.
But BD is equal to BA, AC. Therefore BA, AC are greater than BC.
PROPOSITION 20. THEOREM
| Any two sides of a triangle are together greater than the third side. | |
| Let ABC be a triangle; | |
| then any two sides of it taken together are greater than the remaining side; namely, | |
| BA, AC are greater than BC; | |
| AB, BC are greater than AC; | |
| BC, CA are greater than AB. | |
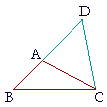 |
|
| Extend BA to D, making AD equal to AC, and draw DC. |
|
| Then, since CA is equal to AD, | (Construction) |
| angle ADC is equal to angle ACD. | (I. 5) |
| Now angle BCD is greater than angle ACD; | (Axiom 5) |
| therefore angle BCD is greater than the equal angle ADC. | |
| In triangle DCB, then, angle DCB is greater than angle BDC; | |
| and the greater angle is opposite the greater side; | (I. 19) |
| therefore, | |
| side BD is greater than side BC. | |
| But BD is equal to BA, AD together, | |
| and AD is equal to AC; | |
| therefore BD is equal to BA, AC together; | |
| therefore BA, AC are greater than BC. | |
| Similarly, we can prove that AB, BC are greater than CA, and BC, CA greater than AB. | |
| Therefore, any two sides etc. Q.E.D. | |
![]()
In other words: A straight line is the shortest distance between two points!
If anyone wanted to ridicule mathematics for its insistence on the axiomatic method of orderly proof, then this theorem offers a wide target. In fact, the Epicureans (those Athenian free-thinkers, who defined philosophy as the art of making life happy), did exactly that. They said that this theorem required no proof and was known even to an ass. For if hay was placed at one vertex and an ass at another, they argued, the poor dumb animal would not travel two sides of the triangle to get his food, but only the one side that separated them.
What scorn the true philosopher must bear! And what can the mathematician do but point out, patiently, that mathematics, as a logical science, relies on deduction from first principles. Those principles moreover are to be as few in number as possible -- whatever can be proved should be. That is the intellectual sport.
Please "turn" the page and do some Problems.
or
Continue on to the next proposition.
Table of Contents | Introduction | Home
Please make a donation to keep TheMathPage online.
Even $1 will help.
Copyright © 2021 Lawrence Spector
Questions or comments?
E-mail: teacher@themathpage.com