skill

S k i l l
 i n
A R I T H M E T I C

Table of Contents | Home | Introduction

Lesson 25  Section 2

SUBTRACTING MIXED NUMBERS

Back to Section 1

  Example 1. 8 4
5		  − 1 1
5

Solution.  In this example, we may simply subtract the whole numbers and subtract the fractions -- similarly to adding mixed numbers.

  Example 1. 8 4
5		  − 1 1
5		  =  7 3
5		 .

But consider the following, in which the fractions are reversed:

  Example 1. 8 1
5		  − 1 4
5		 .
How can we deal with that?  We cannot take  4
5		  from  1
5		 .

To see how to deal with it, consider the following:

add mixed units

We cannot take 40 minutes from 10 minutes -- we need more minutes. To get them, we will break off 1 of the 7 hours, and decompose it into 60 minutes.  We then regroup them with 10 minutes.

60 minutes + 10 minutes = 70 minutes:

add mixed units

2 hours from 6 hours is 4 hours.  40 minutes from 70 minutes is 30 minutes.

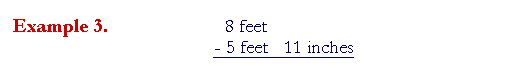
add mixed units

Solution.  We cannot take 11 inches from no inches.  To make inches, then, from 8 feet we will take 1 foot -- which is 12 inches:

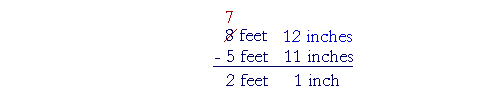
add mixed units

5 feet from 7 feet is 2 feet.  11 inches from 12 inches is 1 inch.

We can now return to our problem:

8 1
5		  − 1 4
5		 .

We need more fifths. Where will we get them?  From 8.  We will break off 1 from 8, and decompose it into five fifths.  (Lesson 21, Example 4.)

add mixed numbers

We will then add those five fifths with the original five fifths making a total of six fifths.

Then:

"1 from 7 is 6.   4
5		  from  6
5		  is  2
5		 ."

*

Actually, the simplest way to do this problem is mentally, by rounding off 1four fifths to 2. (Compare Lesson 7.) That is, add one fifths to both numbers.

8one fifths − 1four fifths = 8one fifths − 2 = 6one fifths.

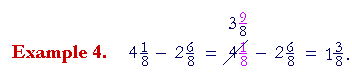
add mixed numbers
Break off 1 from 4.  Express it as  8
8		 , and add it to  1
8		 :

Now the mystery, if any, is:  How does that numerator get to be 9?

9 is the sum of the original numerator 1 and denominator 8:

1/8 + 8/8 = 9/8
  Example 5.   Write the missing numerator:  9 2
5		   = 8 ?
5
  Answer.   8 7
5

7 is the sum of denominator plus numerator:  5 + 2.

  Example 6.      9 2
7		  − 3 5
7		   = ?
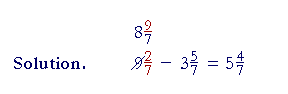
add mixed numbers
9 2
7		  becomes 8 9
7		 .  The improper numerator 9 is the sum of the
  denominator and numerator of   2
7		 :   7 + 2 = 9.
  Example 7. 6 − 1 2
3		   =   5 3
3		  − 1 2
3
 
    =  4 1
3		 .
"1 from 5 is 4.    2
3		  from  3
3		  is  1
3		 ."

Again, the simplest way to do this is mentally by rounding off 1one fifths to 2.  Add one third. (Lesson 21.)

The problem then becomes

6 − 1one third = 6one third − 2 = 4one third.

  Example 8.   Prove:   6 − 1 2
3		  = 4 1
3

Solution.   According to the meaning of subtraction,

1 2
3		  + 4 1
3		  = 5 3
3		  = 6.

Compare Lesson 7, Example 2.

  Example 9.    6 1
2		  − 2 3
4

Solution.  First, we must make the denominators the same:

  Example 10.     6 1
2		  − 2 3
4		  = 6 2
4		  − 2 3
4

We cannot take from ; therefore, on taking —1—from 6, the fraction becomes 4-fourths + 2 -fourths = 6-fourths:

  Example 9.     6 1
2		  − 2 3
4		  = 5 6
4		  − 2 3
4
   = 3 3
4		 .  
"2 from 5 is 3.   3
4		  from  6
4		  is  3
4		 ."

Mentally:

6 1
2		  − 2 3
4		 .
 
"3 from 6 1
2		  is 3 1
2
 
Plus   1
4		  is 3 3
4		 ."

Please "turn" the page and do some Problems.

or

Continue on to the next Lesson.

Previous Section

Introduction | Home | Table of Contents

Copyright © 2021 Lawrence Spector

Questions or comments?

E-mail:  teacher@themathpage.com